Adaptive filters are widely used in communication, active noise control (ANC), and acoustic echo cancellation (AEC). Subband adaptive filters (SAF) are commonly employed to improve the convergence behavior. However, the convergence rate of the traditional subband adaptive filters is reduced due to aliasing and band-edge effects.
To address this problem, in the year of 2012, researchers from the Institute of Acoustics (IOA) of the Chinese Academy of Sciences had considered an improved multiband-structured subband adaptive filter (IMSAF) algorithm as shown in Fig. 1.
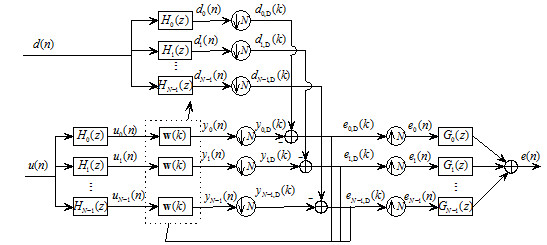
Fig. 1 Block diagram of the IMSAF algorithm (Image by IOA).
When the projection order and/or the number of subbands are increased, the convergence rate of IMSAF improves at the cost of increased complexity. As a result, researchers from the IOA have proposed several approaches to reduce the complexity of IMSAF throughout this year of 2015.
It turns out that the complexity of IMSAF can be reduced to O(2M) from the original O(2PM) operations per sample (M being the length of adaptive filter and P being the projection order). Besides, they also applied the method to a family of AP algorithms (DCD-AP, APS, MFxAP and MPAF) and solved the fast exact implementation problems of these algorithms.
Sequentially, the transient and steady-state behaviors of IMSAF have been researched in theory. Analysis does not require a model for the input signal. Theoretical analysis of the IMSAF algorithm is in good agreement with computer simulation results as shown in Fig. 2.
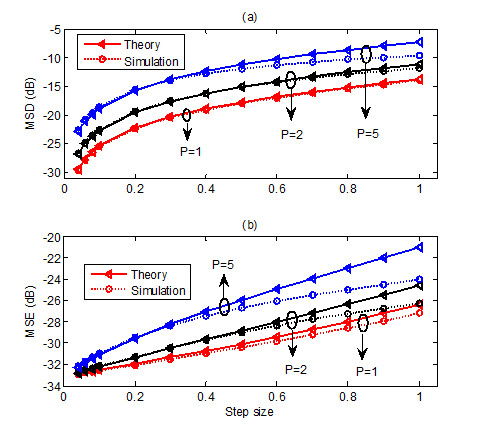
Fig. 2 Steady-state MSD and MSE curves of the standard IMSAF algorithm, with M=36,N=4 and P=1,2,5, SNR=30 dB. The input signal is an AR(10) process (Image by IOA).
Through sequential research by researchers from the IOA, research of the IMSAF algorithm gains ground.
There are four classical time-domain adaptive filtering algorithms, namely least-mean-square (LMS), normalized LMS (NLMS), affine projection (AP) and recursive least squares (RLS). The LMS has the lowest complexity but also the poorest performance, while the RLS has the highest complexity but best performance.
Research results show that the proposed IMSAF algorithm can set up a bridge between AP and RLS as shown in Fig. 3. That means the convergence rate of IMSAF is somewhat slower than that of RLS but much faster than that of AP as shown in Fig. 4.
However, the complexity of the proposed IMSAF can be comparable to fast AP if the fast approaches are used. Moreover, the IMSAF exhibits good numerical stability which is preferable for practice. The IMSAF algorithm is appealing for application in various fields such as AEC/ANC given its favorable properties.
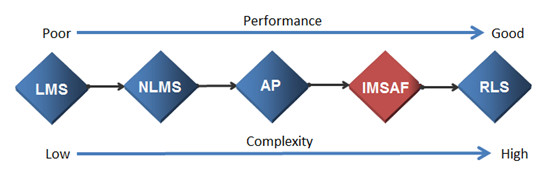
Fig. 3 Relationship between several adaptive filtering algorithms (Image by IOA).
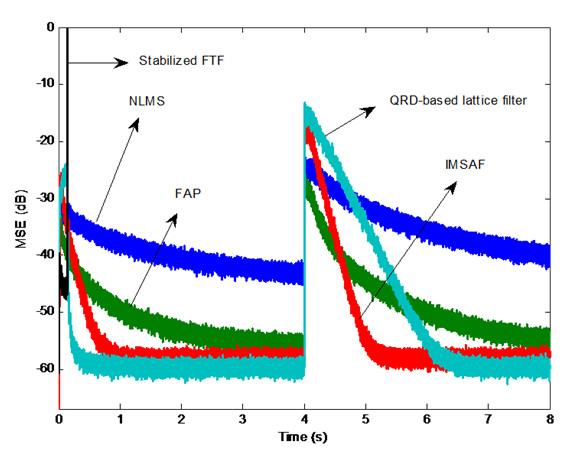
Fig. 4 MSE curves of the NLMS, FAP, IMSAF, QRD-based lattice filter and stabilized FTF algorithms with an AR(10) process as input; M=1024 and SNR=40 dB (Image by IOA).
References:
[1] F. Yang, M. Wu, P. Ji, and J. Yang, "An improved multiband-structured subband adaptive filter algorithm," IEEE Signal Process. Lett., vol. 19, no. 10, pp.647–650, Oct. 2012.
[2] F. Yang, M. Wu, P. Ji, and J. Yang, "Low-complexity implementation of the improved multiband-structured subband adaptive filter algorithm," IEEE Trans. Signal Process., vol. 63, no. 19, pp. 5133–5148, Oct. 2015.
[3] F. Yang, M. Wu, J. Yang, and Z. Kuang, "A fast exact filtering approach to a family of affine projection-type algorithms," Signal Process., vol.101, pp. 1–10, Aug. 2014.
[4] F. Yang, and J. Yang, "Fast implementation of a family of memory proportionate affine projection algorithm," Proc. IEEE ICASSP, April, 2015, pp. 3507-3511.
[5] F. Yang, M. Wu, P. Ji, Z. Kuang, and J. Yang, "Transient and steady-state analyses of the improved multiband-structured subband adaptive filter algorithm," IET Signal Process., in press.
Contact:
YANG Feiran
Key Laboratory of Noise and Vibration Research, Institute of Acoustics, Chinese Academy of Sciences, 100190 Beijing, China
Email:feiran@mail.ioa.ac.cn


