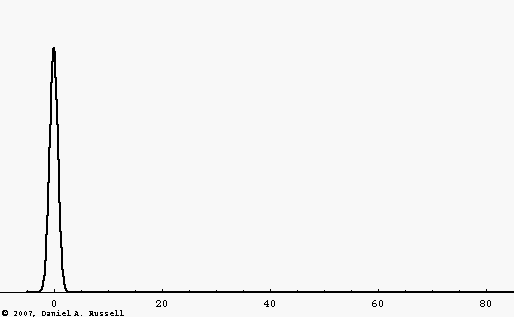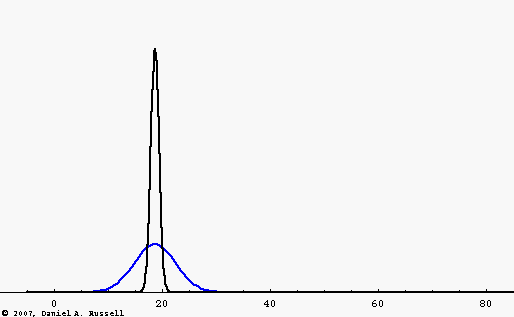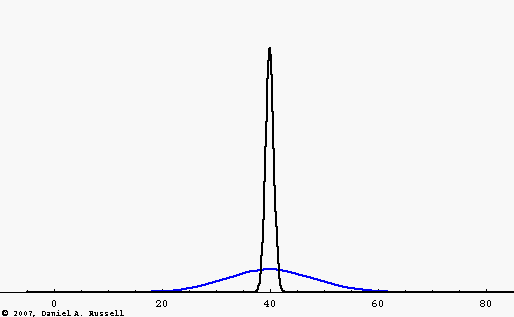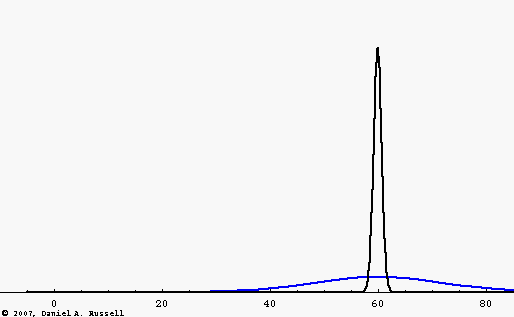If the wave speed dependsonlyon the physical properties of the medium (i.e., the elastic and inertia properties of a mechanical medium, or the relative permeability and permittivity for EM waves) then the wave speed is a constant, independent of frequency. Such a medium is called anon-dispersivemedium and waves traveling through this medium will maintain a constant shape. This is illustrated by theblack wave pulsein the animation at left. The pulse is a Gaussian function, containing multiple frequencies. Since the wave speed is constant, all frequencies travel at the same speed and the pulse maintains constant shape.

However, there are many examples ofdispersivemedia where, for various reasons, the wave speed depends on the frequency of the wave. The blue wave pulse in the animation is the same Gaussian function as the black pulse, consisting of a large number of frequency components added together. However, now the wave speed depends on frequency, with higher frequencies traveling faster than lower frequencies. As a result, the wave pulse spreads out and changes shape as it travels.

The "middles" of both wave pulses travel at the same speed. This fact leads to the concept of a "group velocity" which is the speed with which the energy carried by the wave travels. The group velocity is the same for each wave pulse.

 The animation at left shows a wave packet built up from a sum of 100 cosine functions with different frequencies. In a non-dispersive medium all of the different frequency components travel at the same speed so the wave function doesn't change at all as it travels.